Course outline: The outline can be downloaded here.
Course playlist: Here is a youtube link to the lecture recordings. Please note these recordings are not professionally done and do not meet highest standards, but they can still be helpful.
Class timings: 3:30 to 4:45 pm, Monday and Wednesday, Room: 204 SSE Complex.
A quick review (1 lecture):
What is light? difference between geometric, physical and quantum optics, quantum description of polarization, Bloch sphere, interferometry with a single photon, states and operators, Maxwell’s equations, Fermat’s principle
Planck’s radiation and quantization of cavity radiation (1 lecture): Density of modes, Rayleigh-Jeans model, Planck’s upgrade, Bose-Einstein distribution, quantization of energy
Absorption and emission of radiation from a classical viewpoint (2 lectures): Einstein’s rate equations, A and B coefficients, spontaneous, stimulated emission, Lorentz’s oscillator dipole model of absorption and emission, spectral lineshapes, broadening mechanisms (natural, pressure, Doppler), radiation damping. I also showed the in-class demo on spectral emissions from discharge lamps. Finally here is the first homework and here is its solution supplied by Asad Asif.
Absorption and emission of radiation from a semiclassical viewpoint (5 lectures): This module treats the atom as a two-level quantum system, we start with an atom weakly coupled to electromagnetic radiation, deciphering the role of the electric dipole operator recovering an estimate for Einstein’s A and B coefficients, followed by the strong coupling regime that gives us Rabi oscillations. We also discussed the role of off-resonance, description in terms of Bloch sphere, role of damping. Finally, we derived and solved the optical Bloch equations, showing key results as we went along. We also touched upon saturation broadening. Here is the pdf of a Mathematica notebook that shows the evolution of coherences and populations derived from the optical Bloch equations. Finally, we discussed saturation, photon scattering rates and its linkage with the phenomenon of absorption. Here is the the second homework to give you some problems to attempt. Here is my solution and here is a a computer code that solves part (f) of Question 4.
Quantization of electromagnetic radiation (5 lectures): Treating the light as a harmonic oscillator starting with single mode fields, field quadratures, electric and magnetic field operators, number (Fock) states and their properties, coherent states and their properties, sqeezed states, the vacuum state, displacement operator, phase diagrams, concept of quantum phase, uncertainty relationships, multi-mode fields (here you can can access my handwritten notes on this topic), thermal states. Attempt this homework. A partial and quick solution is here.
Mid Term: Here is midterm exam whose tentative solution can be accessed here.
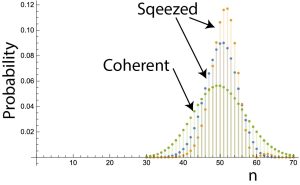
Photon detection statistics and correlations (8 lectures): Photon statistics for coherent, chaotic, thermal and quantum light, Poissonian, super-Poissonian and sub-Poissonian statistics, relation between photons and photon counts, squeezed states and their average values, noise in squeezed states. There will be two homeworks on these topics. Here is the first homework on this topic and fourth in this course. Finally, here is the second homework on this topic and the fifth in this course with a tentative solution supplied herewith. This last homework can show you that squeezed states are nonclassical and give more a regular photon statistics than the most uniform light that is possible to envisage in the classical world (called the coherent state).
Photon bunching and antibunching (3 lectures): The second-order correlation function g2, action of beamsplitter and interferometer, quantum interferometry, bunching and antibunching of photons, g2 for coherent light, chaotic light and number states.
Quantum atom-photon interactions (4 lectures): This set of lectures revolves around fully quantum-mechanical atom-photon interactions. The Jaynes-Cummings Hamiltonian is discussed, concepts of bare and dressed states, and the joint evolution of atom-photon states is sketched. Other concepts that we deal with in these lectures include the interaction of a two-level atom with coherent field, number states and the vacuum state, showing vacuum Rabi oscillations and the underlying principle of reversible spontaneous emission. The final lecture styled as a seminar discussed circuit-QED (quantum electrodynamics) with an elaboration of how the superconducting transmon can act as a qubit on quantum computing platforms. The details of the Jaynes-Cummings models covered in this section are fully covered in Matteo Bina’s tutorial while articles published in PRX Quantum and Applied Physics Reviews provide the interested readers with more details on building superconducting quantum devices. Please download homework no. 6 and here is its partial solution.
The long awaited take-home final exam.