This course is intended to be a first introduction to quantum phenomena in nature. Quantum Mechanics forms the basis of our description of nature at small scales and a clear understanding of it is required to understand phenomena ranging from atoms and chemical bonding to semiconductors and nuclear physics. We will present a concise and comprehensive picture of quantum theory with emphasis on concept building. The concepts will be organized around the ideas of wave particle duality, uncertainty, superposition, entanglement, and their physical and conceptual consequences. Numerous applications to real world phenomena will be discussed throughout the course. The course also has a component that discusses the application of statistical ideas in physics and this leads to concepts of irreversibility, entropy and the energy flow inside the universe.
Instructor: [Dr. Muhammad Sabieh Anwar 
]
Course outline: Please find here.
Class timings: 2:00 to 3:50 pm, Tuesday and Thursday, Room: SSE_B-92, basement of SSE.
Office hours: 4:30 to 5:30 pm, Tuesday and Thursday, Room: 9-103A, Feynman wing (Dr. Sabieh’s office).
Teaching assistants: Shirin Abbas, Diya Batool and Salman Shahid
TA Office Hours:
Diya: Monday and Wednesday, 3-4 p.m.
Shireen: Monday and Wednesday, 11-12 p.m.
Salman: Tuesday and Thursday, 12.30-1.30 p.m. and Thursday 3.20-4.20 p.m
Diya and Shireen will be available in the Physics Lobby (SSE, first floor, Feynman Wing, general sitting area). Salman will be available in the SA Computer Lab.
Supplementary texts: Quantum Mechanics for Beginners (QMB) by Dr. Suhail Zubairy, Oxford University Press; Nonclassical Physics: Beyond Newton’s View by Randy Harris (RH). None of these books provides a complete one-to-one map with the course progression. But both can be extremely useful. I do recommend reading QMB: Chapter 5 to 9.
A nice book which has the same coverage of topics (almost) as our course is the Six Quantum Pieces by Valerio Scarani.
Jonathan Jones’s and Dieter’s Quantum Information, Computation and Communication (JJ) is also an excellent resource.
You can also refer to my unprocessed notes (MSA); but mind you, these are still unprocessed notes.
Previous offerings: Here is the weblink 
for the same course I taught in Spring 2013 and here is it for 2018. And here is the weblink for the offering in 2009 and weblink for the offering in 2011 with all the video recordings, assignments, exams and their solutions.
This is an evolving course. The grading scheme is as follows
| Homeworks (4) | 0% |
| Quizzes (4, all count) | 30% |
| Midterm (one) | 30% |
| Final exam (one) | 40% |
I have the liberty to vary the scheme by +10% or -10%.
Course Progression:
.
| 1. | Basic tenets of quantum physics (the big idea): superposition, entanglement, measurement, quantization, uncertainty. (If you want a good review on the big picture and like to read, I would recommend Quantum Theory: A Very Short Introduction by John Polkinghorne.)
Complex numbers |
| 2. | Quantum states (first lecture): physical implementations of the qubit (spin of an electron, polarization of a photon, path of a photon). (MSA Ch. 5) |
| 3. | Quantum states (second lecture): basis states, ket representation, quantum interferometer, describing probabilities of outcomes. (QMB Ch. 9) |
| 4. | Wave-particle Duality, Uncertainty Principle, Born’s Postulate, the Mach-Zehnder Interferometer. (QMB 5.2-5.4, Ch. 7, Ch. 8) (Mach Zehnder Simulation) |
| 5. | Interaction-free measurement, quantum interferometry with a Mach Zehnder interferometer, some ideas emanating from experiments with photon polarization and the measurement postulate, Stern Gerlach interferometry. (Interaction Free Measurement) |
| 6. | Looking at the polarization degree of freedom, measurement postulate, describing Stern-Gerlach experiment, composite systems, quantum erasure. |
| 7. | Quiz: Group 1 Solution , Group 2 Solution |
| 8. | Quantum erasure, delayed choice experiments, interference experiments with electrons, first introduction to composite systems. (QMB Ch. 8 and 9). Also see the first three chapters of Feynman’s lectures in Physics (vol. 3). Also read this article: Teaching Quantum Theory in the Introductory Course by Art Hobson. |
| 9. | Vector representations of states (kets) and their duals (bras), unitary matrices as operators (Valerio Ch. 6), quantum circuit model, quantum logic gates (QMB Ch. 16), Zeno effect, the rotation matrix. As we start going deeper into quantum information, this article by Popescu and DiVincenzo will be an ultimately illuminating read. Here is a Youtube playlist comprising my eight lectures on quantum computing fundamentals. They will come in really useful for this course, hopefully! |
| 10. | Two-qubit gates, a really simple quantum algorithm and meaning of quantum computers, (the impossible) quantum cloning, entanglement and quantum teleportation. See Ch. 5 of my unprocessed notes, and also Ch. 1 and 5 from Jonathan Jones’s book. |
| 11. | Quantum entanglement, quantum no-cloning. |
| 12. | Quantum teleportation, entanglement swapping, quantum superdense coding, nonlocality, Bell’s inequalities and Tsirelson bound. Lectures 11 and 12 are adequately covered in Scarani’s Chapters 3, 4 and 5. |
| 13. | Equation of motion, Hamilton’s equations, solving a differential equation, time-dependent Schrodinger equation and its solution, Hamiltonian operator, Pauli gates. |
| 14. | Presentation, Quiz 2 Solution Group 1, Quiz 2 Solution Group 2 |
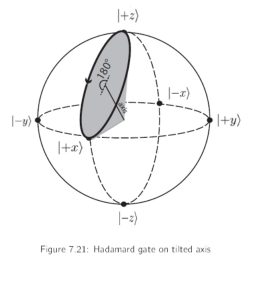
| 15. | The Bloch sphere, quantum gates as rotations on the Bloch sphere, magnetic resonance (spin-1/2 inside a magnetic field that switches on and off), precession, implementing NOT, Hadamard gates. For lectures 15, 17 and 18, read Chapter 3 of JJ.
|
| 16. | Midterm Group 1 Solution, Midterm Group 2 Solution |
| 17. | Some application of time evolution, rotations on a Bloch sphere (continued), Rabi flopping, NMR spectroscopy, Ramsey interferometry, the idea of composite rotations. Here is the link to a Python notebook that you can access to visualize rotations on the Bloch sphere. |
| 18. | The concept of the wave function, plane waves, free particle, Gaussian wavepackets, motivating the time-independent Schrodinger equation. The wavefunction concept covered in Ch. 18 and exemplified through the contents of lecture 19 are well represented in Chapters 3 and 4 of RH. |
| 19. | The infinite well, energy eigenstates and their time evolution. |
| 20. | Reflection and transmission from a potential step, graphical method to draw wavefunctions, Bohr’s correspondence principle, quantum tunneling, radioactivity, some aspects of nuclear energy levels. |
| 21. | Quiz 3 Group 1 Solution, Quiz 3 Group 2 Solution |
| 22. | Infrared absorption spectroscopy and basic themes of spectroscopy, vibrational levels, 3-dimensional infinite well and a demonstration on energy quantization and spectroscopy from discharge tubes. |
| 23. | Wavefunctions for the hydrogen atom, symmetric potential, radial and angular parts, explicit calculation of the azimuthal part, plotting the orbitals. RH Sections 6.1 to 6.9 are a must read for this lecture and the one that follows. Here is a listing of orbitals and their wavefunctions. This sheet will be provided to you in exams too. A very nice visualizations of orbitals and other wavefunctions can be seen on this page. Here is also a detailed solution to the angular part of the Schrodinger equation |
| 24. | Drawing orbitals, radial probability densities, getting probabilities from wavefunctions, quantization of energy, angular momentum and its components. |
| 25. | Quantization of energy, angular momentum’s modulus and its projection along one axis, transitions are explained by superpositions, the simple concept of a photon. |
| 26. | Quiz 4 Group 1 Solution, Quiz 4 Group 2 |
Homeworks and miscellaneous assessments
| HW 1 and its solution
First practice problem and its solution Second problem set (a compilation including most solutions) |
HW 3 and its solution
HW 4 and its solution (NEW!) |